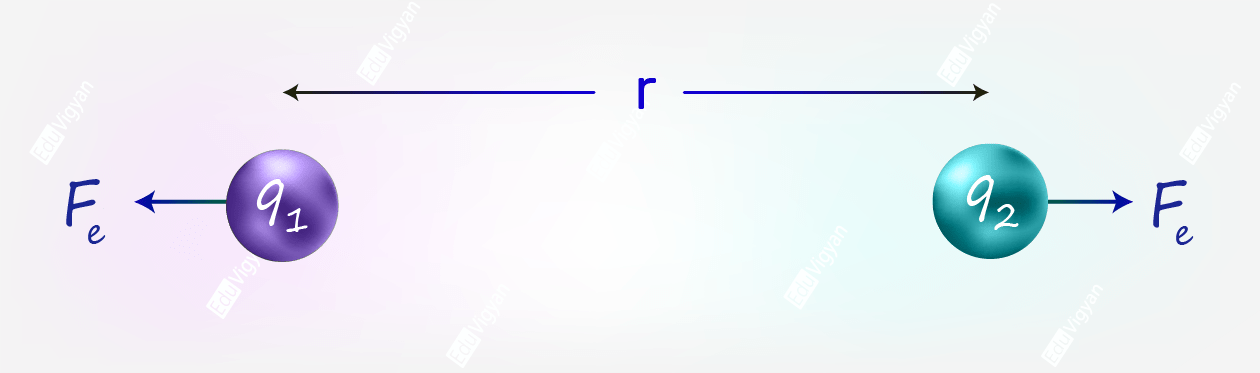
The Coulomb’s Law of Electrostatic Force gives the force between two stationary charged particles. It states that
the force between two charged particles is directly proportional to the multiplication of the charges and inversely proportional to the square of the distance between them.
According to this law, the magnitude of the electrostatic force between two point-charged particles q_1 and q_2 is given by;
F_e = \dfrac{kq_1q_2}{r^2}
where r is the distance between q_1 and q_2, and k is a proportionality constant.
The constant k is given by;
k = \dfrac{1}{4 \pi \epsilon}
where \epsilon is the permittivity of the medium in which the charged particles are placed.
The permittivity of vacuum is denoted by \epsilon_0 and its value is found to be;
\epsilon_0=8.854\times10^{ -12}\rm \ C^2/Nm^2
So, if the charged particles are placed in a vacuum medium then the constant k can be calculated as;
k = \dfrac{1}{4 \pi \epsilon_0} \approx9 \times10^9\ \rm Nm^2/C^2
The nature of electrostatic force between two charged particles:
- Like charges repel each other, and
- unlike charges attract each other.