QUESTION:
Two identical vessels are connected by a tube with a valve letting the gas pass from one vessel into the other if the pressure difference \Delta P \geq 1.10\rm \ atm. Initially, there was a vacuum in one vessel while the other contained ideal gas at a temperature T_1 = 27 \rm \ ^0C and pressure P_1 = 1.00\rm \ atm. Then both vessels were heated to a temperature T_2 =1 07\rm \ ^0C. Up to what value will the pressure in the first vessel (which had vacuum initially) increase?
To solve this problem, you must have an idea of the ideal gas law.
SOLUTION:
After the valve is opened and heating started, some gas passes into the evacuated vessel (vessel 1) till pressure difference becomes \Delta P.
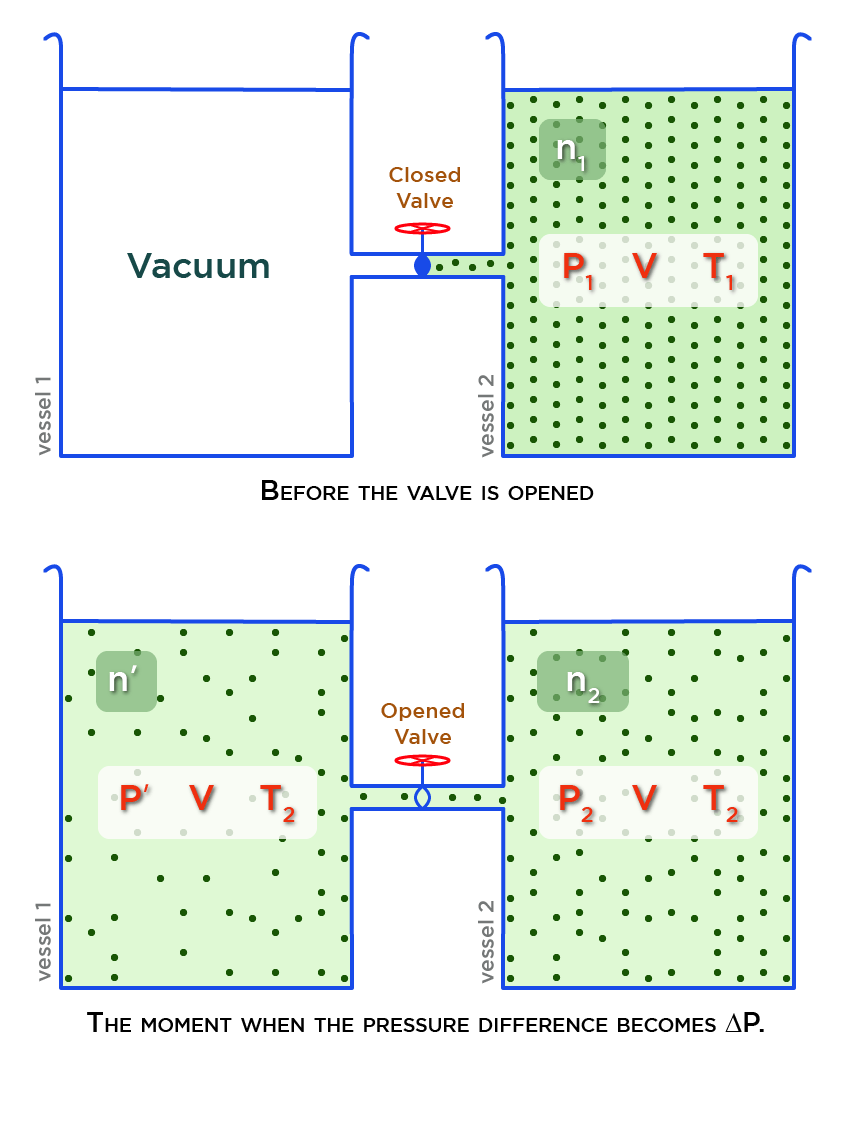
Let’s suppose
- n_1 is the number of mole of the gas enclosed in the vessel 2 before the valve is opened,
- v is the volume of each vessel,
- P_1, and T_1 are the pressure and temperature of the gas in the vessel 2 before the valve is opened,
- P_2 is the pressure of the gas in the vessel 2 when the pressure difference becomes \Delta P after the valve is opened,
- T_2 is the common temperature of the gas in vessel 1 and vessel 2 when the pressure difference becomes \Delta P after the valve is opened,
- P^\prime is the pressure of the gas in the vessel 1 when the pressure difference becomes \Delta P after the valve is opened,
- n_2 is the number of mole of the gas enclosed in vessel 2 when the pressure difference becomes \Delta P.
- n^\prime = n_1-n_2 is the number of mole of the gas enclosed in vessel 1 when the pressure difference becomes \Delta P.
Given:
- \Delta P \geq 1.10\rm \ atm
- T_1 = 27\rm \ ^0C = 273 + 27\rm \ K = 300\rm \ K
- P_1 = 1.00\rm \ atm
- T_2 =107\rm \ ^0C = 273 + 107\rm \ K = 380\rm \ K
From the ideal gas equation, we have
\begin{aligned}P_1V&=n_1RT_1 \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ (1)\\\end{aligned}
\begin{aligned}P_2V &= n_2RT_2 \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ \ (2)\\\end{aligned}
\begin{aligned}P^\prime V &= n^\prime RT_2\\ &= (n_1-n_2)RT_2 \ \ \ \ \ \ \ \ \ \ \ \ \ (3)\\\end{aligned}
From equations 1, 2 and 3
\begin{aligned}P^\prime V &= \left ( \dfrac{P_1V}{RT_1}-\dfrac{P_2V}{RT_2}\right )RT_2\\\Rightarrow P^\prime &=\left ( \dfrac{P_1}{T_1}-\dfrac{P_2}{T_2}\right )T_2\\\end{aligned}
But, we have P_2-P^\prime = \Delta P \Rightarrow P_2 = P^\prime+ \Delta P
So,
\begin{aligned} P^\prime &=\left ( \dfrac{P_1}{T_1}-\dfrac{P_2}{T_2}\right ) T_2\\&=\left ( \dfrac{P_1}{T_1}-\dfrac{ P^\prime+ \Delta P }{T_2}\right ) T_2\\&= \dfrac{P_1T_2}{T_1}-P^\prime -\Delta P\\\Rightarrow P^\prime&= \dfrac{1}{2} \left ( \dfrac{P_1T_2}{T_1}-\Delta P \right ) \\&= \dfrac{1}{2} \left ( \dfrac{(1.00\rm \ atm)( 380\rm \ K)}{( 300\rm \ K)}- 1.10\rm \ atm \right )\\&= 0.08\rm \ atm \\\end{aligned}
Therefore, the pressure in the first vessel up to which it increases is
P^\prime = 0.08\rm \ atm




