QUESTION:
A uniform solid sphere of radius r starts rolling down without slipping from the top of another fixed sphere of radius R. Find the angular velocity of the sphere of radius r at the instant when it leaves contact with the surface of the fixed sphere.
Please Click Here for the concept behind this problem.
SOLUTION:
Given:
The radius of the small sphere is r, and
The radius of the fixed sphere is R.
The free-body diagram of the small sphere when it leaves contact with the surface of the fixed sphere is shown in the diagram.
Here,
- mg is the weight of the small sphere, and
- F_c is the centrifugal force acting on the small sphere.
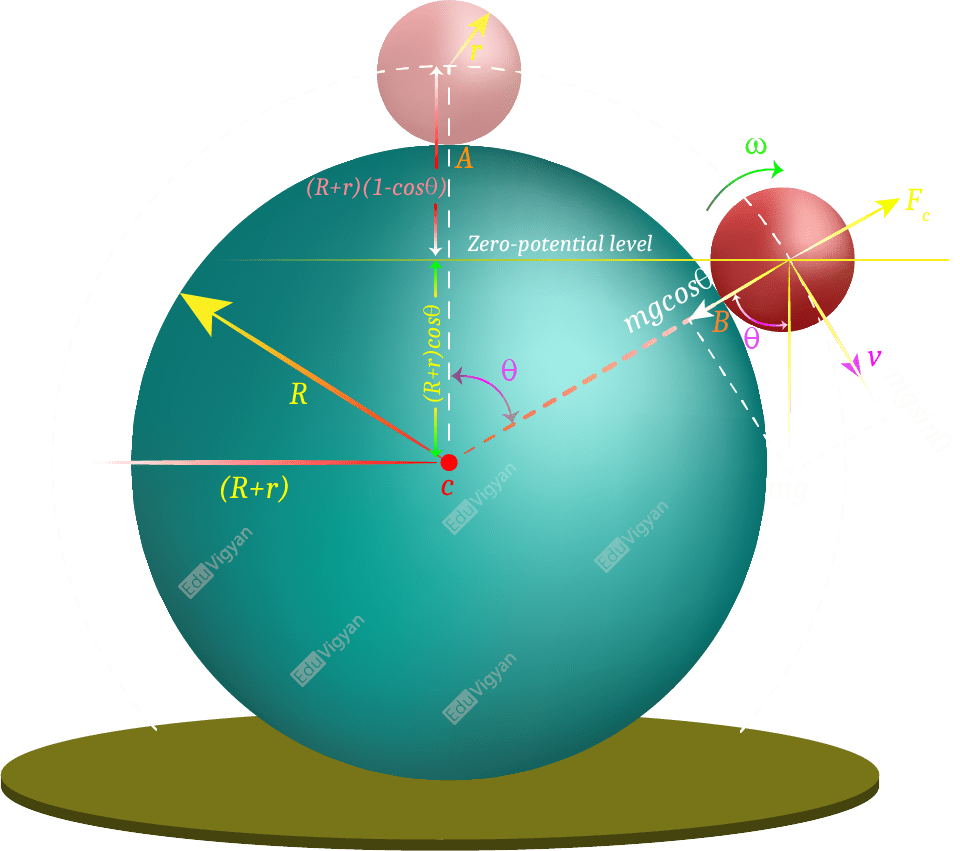
Free-Body Diagram Of The Small Sphere:
If the small sphere leaves contact with the surface of the fixed sphere at point B then we have;
\begin{aligned}F_c &= mg\cos\theta\\\Rightarrow \dfrac{mv^2}{(R+r)} &= mg\cos\theta\\\Rightarrow \dfrac{v^2}{(R+r)} &= g\cos\theta\end{aligned}
As the small sphere rolling without slipping so we have;
v = \omega R
The momentum of inertia of the small sphere about its own axis is;
I = \dfrac{2}{5}mr^2
The kinetic energy of the small sphere at point A is;
K.E._{A}=0
Potential energy of the small sphere at point A is;
P.E._{A} =mgh= mg(R+r)(1-\cos\theta)
The kinetic energy of the small sphere at point B is;
K.E._{B}=\dfrac{1}{2}mv ^2+\dfrac{1}{2}I\omega^2
Potential energy of the small sphere at point B is;
P.E._{B} = 0
From the conservation of mechanical energy, the mechanical energy of the small sphere at point A is equal to the mechanical energy of the small sphere at point B.
\begin{aligned}K.E._{A}+P.E._{A} &= K.E._{B}+P.E._{B} \\\Rightarrow 0+mg(R+r)(1-\cos\theta) &= (\dfrac{1}{2}mv ^2+\dfrac{1}{2}I\omega ^2)+0\\\Rightarrow mg(R+r)(1-\cos\theta) &= \dfrac{1}{2}mv ^2+\dfrac{1}{2}\times(\dfrac{2}{5}mr^2)\times(\dfrac{v }{r})^2\\\Rightarrow g(R+r)(1-\cos\theta)&=\dfrac{7}{10}v ^2\end{aligned}
From equations (1) and (2), we have;
v = \sqrt{\dfrac{10(R+r)g}{17}}
Therefore, the angular velocity of the small sphere at the instant when it leaves contact with the surface of the fixed sphere is;
\begin{aligned}\omega &=\dfrac{v}{r}\\&=\sqrt{\dfrac{10(R+r)g}{17r^2}}\end{aligned}
(1)
(2)






